The history of the Sith Order encompasses a plethora of plans devised by Lords and Ladies of the Sith across the millennia with the goal of taking control of and reshaping the galaxy according to their ideals.1-4 Among these, Karness Muur, a fallen Jedi and one of the first Sith Lords, conceived and engineered a disease called the “rakghoul plague” as an instrument for seizing power.5,6 The basic characteristics and modes of transmission of this disease were detailed in a document known as the Codex of Karness Muur.5
In particular, the original mode of transmission of the plague involved Sith magic infused into an amulet, the Muur Talisman.5,6 When wearing this amulet, Karness Muur was able to transform most nearby humans into rakghouls — mentally zombie-like creatures, but not physically undead — who obeyed his every order. Rakghouls were highly aggressive carnivores that targeted humans as their primary prey, but would also engage in cannibalism when humans were rare.6 To avoid a reduction in rakghoul numbers or even self-extinction through cannibalism, the wielder of the talisman could direct its power to suppress cannibalistic behaviour. With an ever-growing army of rakghouls at his disposal, Karness Muur planned to assume control of planetary systems and eventually the entire galaxy. Despite the high efficacy of the talisman, Force-sensitive humans and other humanoid species appeared to be immune to its power,5 making the number of potentially susceptible hosts much lower than Karness Muur desired.
To overcome this limitation, the Sith Lord engineered an infectious agent that could be transmitted from a rakghoul to a healthy individual, including Force-sensitive entities and non-human humanoids, through wounds caused by a scratch or a bite.5,6 The infectious agent triggers the metamorphosis of the new host into a rakghoul within a few hours, accompanied by severe pain and bleeding from the conjunctiva, oral mucosa, and skin.6 The disease also rapidly affects the overall body morphology through a number of processes, including the apoptosis of particular finger and toe tissues, the development of claws, and the enlargement of teeth.5,6
Although the Muur Talisman was destroyed by Cade Skywalker 137 years after the Battle of Yavin,6 information on surviving rakghoul populations, on the location of the Codex of Karness Muur, or on amulets with similar properties is not available. A rakghoul invasion of Earth from a galaxy far, far away therefore remains possible, albeit unlikely. In order to design an effective policy for responding to an outbreak of the rakghoul plague, it is imperative to first develop a theoretical understanding of the population dynamics of rakghouls and humans. As currently available models, including those designed for zombie outbreaks,7-10 cannot adequately capture the complex interactions between rakghoul and human individuals, nor those between rakghoul individuals and members of their own species, we developed the first mathematical model of the rakghoul plague. In this article, we explore the behaviour of the model, and obtain realistic predictions of the outcome of the plague, using New South Wales as a model site. Finally, we use the resulting predictions to formulate guidelines for combating and eliminating outbreaks of the rakghoul plague in Australia.
Mathematical model of the population dynamics of humans and rakghouls
To simulate the population dynamics of humans and rakghouls, we developed a model based on the Lotka–Volterra system of ordinary differential equations11 (we refer the curious reader to the online Appendix for full details of the model and its parameters). The model tracks changes in human and rakghoul populations and accounts for their complex interactions. If a human encounters a rakghoul, one of two outcomes is overwhelmingly likely: either the human is killed, or is scratched or bitten (very few individuals have been reported to have escaped an encounter with a rakghoul5,6). In the latter case, the human is then infected with the rakghoul plague and is transformed into a rakghoul within a negligible amount of time; as a result, the human population size is reduced. Rakghoul populations benefit from both killing and infecting humans, but whenever there are few humans around, they become cannibalistic, thus reducing their own population size.
We simulated a hypothetical rakghoul invasion in New South Wales, Australia. The growth rate of the human population (difference between birth and death rates) and the initial population size for humans were based on official 2016 statistics for New South Wales.12,13 However, the scientific literature is scant on details and data regarding human–rakghoul systems; for this reason, we resorted to a combination of common biological sense and insights from only approximately analogous systems (eg, elks and wolves; see online Appendix) to parameterise our mathematical model.
Examples of the different types of resulting dynamics are depicted in Box 1. The most influential parameter of the model appears to be the time taken by a rakghoul to consume killed prey. The strong correlation in the times to extinction of humans and rakghouls is noteworthy, suggesting that taking advantage of the cannibalistic tendencies of rakghouls alone could potentially be a sufficient strategy for combating the plague.
A variant of the model in which humans are evacuated
We also investigated the feasibility of evacuating the human population as a disease control strategy. To evaluate this option, it is key to obtain estimates of the time required for rakghouls to be driven to extinction through cannibalism in the absence of their primary prey. The analysis of the population trajectories revealed a very weak positive correlation between the initial rakghoul population size and the time until cannibalism-mediated extinction (Box 2 and online Appendix), meaning that cannibalism poses a large threat to rakghoul survival. Extinction times varied from a few hours to more than 2.5 days; a human evacuation strategy for eliminating the disease therefore seems very promising.
Extending the original model to include military action
An alternative strategy for disease control and elimination involves the active extermination of disease hosts by military intervention in the form of armed melee combat, further containing rakghoul population growth. We assumed that when a soldier first detects a rakghoul, the latter will always be killed without inflicting any injury on the soldier. Estimates of parameters for the military intervention (such as the proportion of the population of soldiers in the human population, which was assumed to be constant) were based on the number of Australian Defence Force employees in the state of New South Wales.14 To make sense of the numerical solutions of this model, especially in light of the large number of parameters, we resorted to machine learning and, more precisely, to the construction of a decision tree (see online Appendix for details). The final topology of the tree is shown in Box 3, panel A. The tree broadly suggests that the extinction of the local human population can be averted with the aid of the military if rakghouls take more than 32.4 minutes to consume killed prey, or if the military responds within 21.2 hours of the rakghoul invasion. Panel B shows that the tree almost always predicts the true outcome, and is therefore very reliable.
Crisis evaluation and proposed guidelines
The theoretical investigation in the previous sections suggests that an outbreak of the rakghoul plague will undoubtedly induce crisis conditions, disrupting the orderly functioning of the local community with its cataclysmic rate of progression. As there is no clinically approved antidote that reverses the rakghoul transformation, we consider infected individuals to be de facto removed from the human population. In the absence of any intervention, the entire local human population will be driven to extinction within hours or a few days at most, so the situation fulfils the criterion for classification as a “major catastrophe” (ie, a minimum of five deaths per 10 000 adults per day).15
It is therefore essential to devise a detailed plan for defending against an outbreak of the rakghoul plague. Based on the outcomes of the three versions of our model, we propose the following guidelines.
Before the outbreak
To lay the foundations of an efficient and successful response to the rakghoul plague, it is essential that citizens are encouraged to familiarise themselves with the potential threat of a rakghoul invasion. In order to swiftly detect cases of the plague, platforms should be established on which citizens can report rakghoul sightings for further investigation by local authorities.15 Examples of such platforms include websites, Twitter accounts, and a four-digit telephone number via which the public can submit information about rakghoul sightings, together with photos or other media content, if supported by the platform. During an outbreak, operators of these platforms would be encouraged to provide information on the status of the invasion, actions being taken, and official advice. The operation of these platforms would be the responsibility of the state, which should allocate public funding and staff for receiving and responding to user input at any time of day or year.
To ensure optimal combat readiness, soldiers should be aware of the characteristics of the rakghoul plague and be trained in melee combat. The current small arms equipment of the Australian Defence Force, such as the enhanced F88 Austeyr rifle,16 is considered adequate for such operations; no further investment in weaponry is required.
For a successful evacuation strategy to be implemented, it is imperative that numerous high security shelters be constructed. Ideally, these would be subterranean and guarded by armed police during an outbreak, both to limit rakghoul access and to provide safety during any bombardment event undertaken as a desperate means for eradicating remaining rakghouls. Further, the shelters should be able to accommodate the entire population of the state (nearly 8 million people in New South Wales), and should be stocked with non-perishable food and water supplies sufficient for a minimum of 5 days. Finally, emergency communication channels should be established between the shelters and the authorities of neighbouring states to facilitate the exchange of information on the status of the outbreak and decisions that need to be made.
During the outbreak
The proposed algorithm for operation during the outbreak is summarised in Box 4. After confirmation of a rakghoul sighting, it is crucial that the entire local defence force takes immediate action to eliminate the threat; to maximise the probability of success, this must be initiated within 21 hours of the rakghoul invasion. While the military intervention is in progress, citizens should be gradually evacuated to nearby shelters, in compliance with the directives of police and health care professionals, who will ensure that shelters are free from individuals infected with the rakghoul plague. During their time of stay in the shelters, citizens should abide by the orders of public servants, whose duties would include the distribution of food and water rations.
The military operation would be considered successful if the rakghoul population is driven to extinction. Following this, careful examination of each shelter should ensure that the disease had not managed to infiltrate any of them. In such an unfortunate scenario, soldiers should execute the infected hosts and monitor the apparently healthy population of the shelter for at least 2 days to rule out the possibility of other cases of infection going undetected. All healthy humans should eventually be released from the shelters.
Should the military intervention fail, a different path of action is proposed. First, any contaminated shelters should be destroyed by order of the local authorities, to preclude the potential release of new rakghoul individuals. Next, neighbouring state authorities should be notified via the emergency communication channel about the status of the emergency. It will fall to them to decide whether they have the capacity to prevent, through any means necessary, migration of the rakghoul population onto their territories. If the rakghouls’ range expansion can be stopped, it should only be a few days at most until they become extinct through cannibalism. Otherwise, bombardment of the areas occupied by rakghouls should be undertaken promptly, until not a single rakghoul is left standing (or breathing). Similar approaches have been used in the past to control large zombie populations,17,18 especially when their migration appears unavoidable. While bombardments would not affect the human shelters, which should be well below the surface, they will have dire consequences for local ecosystems and infrastructure, and should only be undertaken as a last resort. Before human evacuation is ended, helicopters equipped with thermal cameras should scan the previously infected areas to confirm the eradication of rakghouls.
Future work
The effectiveness of a rakghoul invasion would probably be greatly enhanced if they acquired the ability to infect non-human organisms on Earth, either through evolution or the inspired contribution of a Sith Lord or Lady. Accordingly, the model could be extended to predict the potential consequences of such a change in circumstances.
Further, our current analyses assume a single founder event, consistent with a chance encounter of a small invading population of rakghouls with planet Earth. However, rakghouls may invade relatively nearby inhabited planets that could act as sources of further invasion events. To account for this, as well as to model the spatial interplanetary and intergalactic dynamics of the rakghoul plague, the theoretical model could be extended to include multiple rakghoul metapopulations.19,20
The assumption that human and rakghoul individuals on Earth are evenly distributed in space should also be examined, as heterogeneity in the distribution of either could have important implications for transmission dynamics. The model could thus be extended to account for spatial heterogeneity on Earth.21,22
Finally, a rakghoul outbreak would severely affect the distribution of people in affected areas, as they would all be relocated to underground shelters. The clustering of numerous individuals in small confined spaces would have important implications for any other ongoing epidemic,15,23,24 such as gastroenteritis or lung infections. A sudden increase in population density in a small area could also lead to the emergence of latent anxiety disorders, such as agoraphobia and panic disorder.15,25 Further work should investigate the consequences of population confinement and possible approaches to alleviating these effects.
Conclusions
The looming threat of an outbreak of the rakghoul plague on Earth has been passed over in silence in the literature. To rectify this, we focused our analyses on understanding the potential impacts of the plague on the state of New South Wales. Through mathematical modelling, we showed that the extinction of the local human population can be prevented by a combined strategy of human evacuation and swift military intervention. Further, the predictions of our mathematical model can inform guidelines for a successful defence against the rakghoul plague. It is our hope that future studies will build on and expand on our work, so that a thorough understanding of the rakghoul plague can be attained, fuelling the development of effective strategies for controlling and minimising its spread.
Box 1 – Examples of the population dynamics predicted by our mathematical model, in the absence of any intervention. A–C: A positive relationship between the time it takes a rakghoul to consume prey and the times for human and rakghoul extinction can be detected. D–F: Conversely, a high efficiency of conversion of consumed humans to new rakghouls leads to relatively shorter extinction times, and vice versa*

* The human silhouette was downloaded from PhyloPic.org; the rakghoul silhouette was drawn by the first author (DGK).
Box 2 – The relationship between the initial number of rakghoul individuals (horizontal axis) and the time to extinction in the absence of humans (vertical axis)*
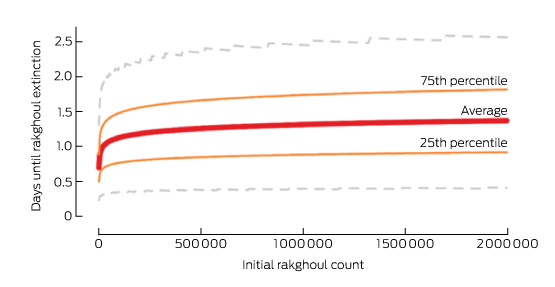
* The thick red line depicts the average of modelling more than 10 000 scenarios for the characteristics of the plague, the two orange lines the scenarios at the 25th and 75th percentiles, whereas the dashed grey lines indicate the two most extreme scenarios.
Box 3 – A. Decision tree for the outcome of the rakghoul plague in the case of a military intervention response by humans. B. Comparison of the true outcome of the rakghoul plague with that predicted by panel A*
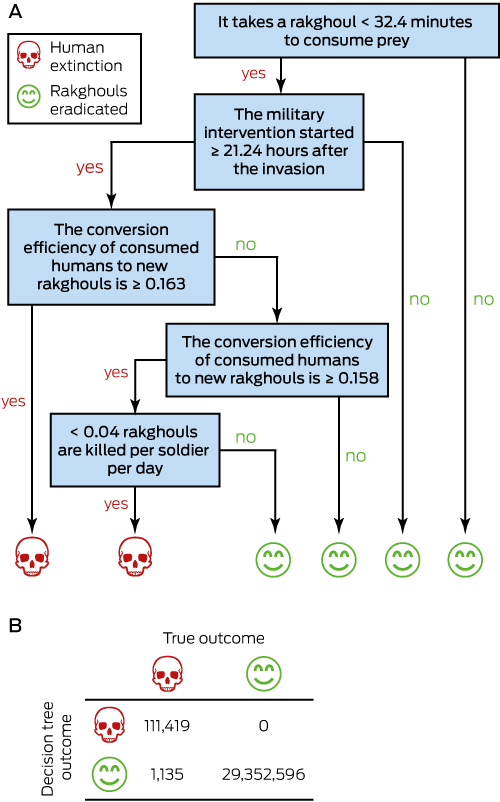
* The subset of the data included in this table was used only to evaluate the performance of the tree and was not part of the training dataset. The table shows that the tree is always accurate when the outcome is rakghoul eradication, whereas it correctly recognises human extinction about 99% of the time.
- Dimitrios-Georgios Kontopoulos1
- Theano Kontopoulou2
- Hsi-Cheng Ho1
- Bernardo García-Carreras1
- 1 Imperial College London, Silwood Park Campus, Ascot, Berkshire, United Kingdom
- 2 Evangelismos Hospital, Athens, Greece
We thank Samraat Pawar for providing comments on an early draft of our article.
No relevant disclosures.
- 1. Freed A, Ross D, McKenna M, et al. Star Wars: the Old Republic. Volume one: Blood of the Empire. Milwaukie (OR): Dark Horse Comics, 2011.
- 2. Stover M, Rood B. The Tenebrous way. Star Wars Insider 2011; (130): 22-29.
- 3. Luceno J. Star Wars: Darth Plagueis. London: Arrow Books, 2012.
- 4. Ostrander J, Duursema J, Dekraker A, et al. Star Wars legends epic collection. Legacy, volume 1. New York (NY): Marvel Worldwide, 2016.
- 5. Miller JJ, Harrison M, Hepburn S, et al. Star Wars: Vector, volume 1. Milwaukie (OR): Dark Horse Comics, 2009.
- 6. Williams R, Ostrander J, Weaver D, et al. Star Wars: Vector, volume 2. Milwaukie (OR): Dark Horse Comics, 2009.
- 7. Munz P, Hudea I, Imad J, Smith? RJ. When zombies attack! Mathematical modelling of an outbreak of zombie infection. In: Tchuenche JM, Chiyaka C, editors. Infectious disease modelling research progress. Hauppauge (NY): Nova Science Publishers, 2009; pp 133-150.
- 8. Witkowski C, Blais B. Bayesian analysis of epidemics — zombies, influenza, and other diseases. arXiv 2013; 1311.6376. https://arxiv.org/abs/1311.6376 (accessed: Sept 2017).
- 9. Smith? RJ. Mathematical modelling of zombies. Ottawa (ON): University of Ottawa Press, 2014.
- 10. Alemi AA, Bierbaum M, Myers CR, Sethna JP. You can run, you can hide: the epidemiology and statistical mechanics of zombies. Phys Rev E 2015; 92: 52801.
- 11. Otto SP, Day T. A biologist’s guide to mathematical modeling in ecology and evolution. Princeton (NJ): Princeton University Press, 2007.
- 12. Australian Bureau of Statistics. 3101.0. Australian demographic statistics, Jun 2016. http://www.abs.gov.au/AUSSTATS/abs@.nsf/allprimarymainfeatures/9D56A542A17EF188CA2580EB001335A8?opendocument (accessed Feb 2017).
- 13. Australian Bureau of Statistics. Deaths, year of registration, summary data, sex, states, territories and Australia. http://stat.data.abs.gov.au/Index.aspx?Queryid=456 (accessed Feb 2017).
- 14. Australian Government, Department of Defence. Defence annual report 2015–16. Volume one: performance, governance and accountability. http://www.defence.gov.au/annualreports/15-16/Downloads/DAR_2015-16_Vol1.pdf (accessed Feb 2017).
- 15. Redmond AD, Mahoney PF, Ryan JM, Macnab C, editors. ABC of conflict and disaster. Malden (MA): Blackwell Publishing, 2006.
- 16. Australian Army. Enhanced F88 [website]. Dec 2016. https://www.army.gov.au/our-work/equipment-and-clothing/small-arms/enhanced-f88 (accessed June 2017).
- 17. O’Bannon D. The return of the living dead [film]. Hemdale Film Corporation, USA, 1985.
- 18. Noussias Y. Evil: In the time of heroes [film]. Audio Visual Enterprises, Greece, 2009.
- 19. Riley S. Large-scale spatial-transmission models of infectious disease. Science 2007; 316: 1298-1301.
- 20. Grenfell B, Harwood J. (Meta)population dynamics of infectious diseases. Trends Ecol Evol 1997; 12: 395-399.
- 21. Lloyd AL, May RM. Spatial heterogeneity in epidemic models. J Theor Biol 1996; 179: 1-11.
- 22. Ostfeld RS, Glass GE, Keesing F. Spatial epidemiology: an emerging (or re-emerging) discipline. Trends Ecol Evol 2005; 20: 328-336.
- 23. Noakes CJ, Beggs CB, Sleigh PA, et al. Modelling the transmission of airborne infections in enclosed spaces. Epidemiol Infect 2006; 134: 1082-1091.
- 24. Sanz J, Xia C-Y, Meloni S, et al. Dynamics of interacting diseases. Phys Rev X 2014; 4: 041005.
- 25. American Psychiatric Association. Diagnostic and statistical manual of mental disorders (DSM-5). Washington (DC): American Psychiatric Publishing, 2013.






Summary
A long time ago in a galaxy far, far away, the Sith Lord Karness Muur engineered the rakghoul plague, a disease that transformed infected humans into near-mindless predatory rakghouls. At its peak, the disease infected millions of individuals, giving rise to armies of rakghouls on a number of planets. Whether rakghoul populations have persisted until this day is not known, making a rakghoul invasion on Earth not completely improbable. Further, a strategy for defence against an outbreak of the disease on Earth has not yet been proposed. To fill this glaring gap, we developed the first mathematical model of the population dynamics of humans and rakghouls during a rakghoul plague outbreak. Using New South Wales as a model site, we then obtained ensembles of model predictions for the outcome of the rakghoul plague in two different disease control strategy scenarios (population evacuation and military intervention), and in the absence thereof. Finally, based on these predictions, we propose a set of policy guidelines for successfully controlling and eliminating outbreaks of the rakghoul plague in Australian states.